Rolle's Theorem and the Mean Value Theorem

Hi everyone! I hope you all had a great weekend. Rolle's Theorem and the Mean Value Theorem are two theorems of Calculus that can seem similar in a way, as they share a common hypothesis. Today, we will explore what these theorems state and examine interesting problems that could be approached with these theorems.

First, let's go over what the theorems state!
1> Rolle's Theorem:
If function f is continuous on the closed interval [a, b], differentiable in the open interval (a, b) and
f(a) = f(b), there exists a number c in (a, b) such that f '(c) = 0.

2> Mean Value Theorem (MVT):
If function f is continuous on the closed interval [a, b] and differentiable in the open interval (a, b), there exists a number c in (a, b) such that f '(c) = {f(b) - f(a)} /( b - a)

Now, let's look at some example problems.

Example 1> Prove that x^3 + x - 1 = 0 has exactly one zero.

Solution> First, we have to use the Intermediate value theorem to prove that there is a zero. For those of us who are not familiar with the Intermediate Value Theorem (IVT), the IVT states that if f is continuous on [a, b] and f(a) < w < f(b), there exists a number c between a and b such that f(c) = w. Let f(x) = x^3 + x - 1. Since f is a polynomial function, it is continuous on its domain (-∞, ∞). Thus f is continuous on [0, 1]. Notice f (0) = -1, f (1) = 1. Thus by the IVT, there exists a number c in (0, 1) such that f (c) = 0. Now, we will use proof by contradiction. Suppose that x^3 + x - 1 has two zeros at a and b. Without loss of generality, let a < b. x^3 + x - 1 is continuous on [0, 1] and since f '(x)= 3x^2 + 1 and this is never undefined, f (x) is differentiable in (0, 1). Since a and b are zeros, f(a) = f(b) = 0. Therefore, by Rolle's Theorem, there exists a number d in (0, 1) such that f '(d) = 0. But, f '(x)= 3x^2 + 1 ≥ 1 and this is a contradiction. Therefore, our hypothesis that f has two zeros were incorrect and therefore we have exactly one zero.

Example 2> Suppose that f(0) = -3 and f '(x) ≤ 5 for all values of x. How large can f (2) possibly be?

Solution> Since f is differentiable in (-∞, ∞), f is continuous in (-∞, ∞), and thus f is continuous on [0, 2]. Therefore, by the Mean Value Theorem, there exists a number c in (0, 2) such that f '(c) = {f(2) - f(0)}/2 = ( f(2)+ 3 )/2 ≤ 5. So, f(2) ≤ 2*5 - 3 = 7. Therefore, the largest f (2) can possibly be is 7.

I just wanted to introduce you all to these theorems and set of questions, because I was intimidated by these proof type questions using these theorems when I saw them. I was confused as of when to use the MVT, IVT, or Rolle's Theorem. Familiarity with the theorems are crucial, and it is important that we remember how to approach each of these problems using the theorems. I hope you all have a great rest of the day and please contact me if you do have any questions.

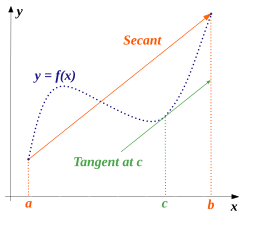Mean Value Theorem; for a given arc between two endpoints,

there is at least one point at which the tangent to the arc is parallel

to the secant through its endpoints.